Chapter 7. Acids, Bases, and Equilibrium

Acids and Bases

From our experience:

Acid = tastes sour

Base = tastes bitter, and feels soapy.

Brønsted-Lowry Acids and Bases

Acid = donates H⁺

Base = accepts H⁺

Using this definition, the following reaction, ammonia reacting with water is:

NH₃	+	H₂O	⇄	NH₄⁺	+	OH^-
Base		Acid		Acid		Base

Notice that the reaction has double-sided arrow, ⇄, to indicate that the reaction can go both direction. This is called equilibrium. It means that all four compound are present in the solution at any given time. Or you can say that not all reactants become products.

In the forward direction, proceeding to right, NH₃ accepted H⁺ to become NH₄⁺, therefore NH₃ is a base, while H₂O donnated H⁺ to NH₃ and H₂O is an acid.

In the reverse direction, the reaction proceeding from right to left, NH₄⁺ is an acid and OH^- is a base.

With the similar argument, we can identify the compound to be acid or base in the following reaction.

HSO₄^-	+	HPO₄^2-	⇄	H₂SO₄^-	+	PO₄^3-
Base		Acid		Acid		Base

Equilibrium

As shown above, equilibrium is a reaction that can go both directions, forward and reverse. And, all compounds in the reaction are present at a given time.

Equilibrium Constant

Even though it can go both ways, the concentration of the compounds are given by the so-called equilibrium constant, K_eq .

For a given reaction,

aA + bB ⇄ cC + dD where a, b, c and d are the stoichiometric coefficients of the balanced chemical equation, then the K_eq is

K_{e q} = \frac{{[C]}^{c} {[D]}^{d}}{{[A]}^{a} {[B]}^{b}}

where [A], [B], etc are the concentration (in molarity) of species A and B in the reaction.

For example, acetic acid is in equilibrium with acetate ion such that

CH₃CO₂H ⇄ H⁺ + CH₃CO₂^- First, according to the Brønsted-Lowry definition of acid-base, acetic acid, CH₃CO₂H, produces H⁺ thus it is an acid. We can write the equilibrium constant for the acid is, then

K_{\begin{array}{l} a c e t i c \\ a c i d \end{array}} = \frac{[H^{+}] [C H_{3} C O_{2}^{-}]}{[C H_{3} C O_{2} H]} = 1.74 \times 10^{- 5}

The numerator terms are the product ions, and the denominator is the reactant. Notice that the value of K_eq is a very small number in acetic acid. It means that the concentration of [H⁺] (and [CH₃CO₂^-) is quite small, and the reactant is in larger concentration.

If [reactant] > [products]	K_eq = small or K_eq < 1
If [reactant] = [products]	K_eq = 1
If [reactant] < [products]	K_eq = large or K_eq > 1

Le Châtelier's Principle

Several factors that upset (or affect) equilibrium.

Adding or subtracting reactants and products

If gas reactions, pressure applied affect the equilibrium

Temperature shifts equilibrium When one of these happens to a reaction, the system tries to return to equilibrium.

Ionization of Water

Even water can ionize! according to the following.

H₂O ⇄ H⁺ + OH^-

K_eq = K_w = 1.0 X 10^-14

As you can see the equilibrium constant in this reaction is very tiny. It means that not too many protons, H⁺, are around in the solution. Remember above that H⁺ represents an acid. So, water is not a very good acid.

The pH Scale

Even though, water is not a very good acid, there are some H⁺ available in water. In order to keep track of relatively small numbers into much more legible way to express, the biochemist Sørensen at Carlsberg Laboratory (associated with the Danish beer company) came up with the pH scale.

p H = - \log [H^{+}]

The pH scale runs from 0 - 14. The range of pH=0 - 7 is acidic and 7 - 14 is basic. Therefore, in the pH scale, more H⁺ is available for a given solution as the value of pH become smaller. The opposite is true that as the value of pH increases less H⁺ is available. The following figure illustrates the relationship between pH and pOH as well as the respective concentrations.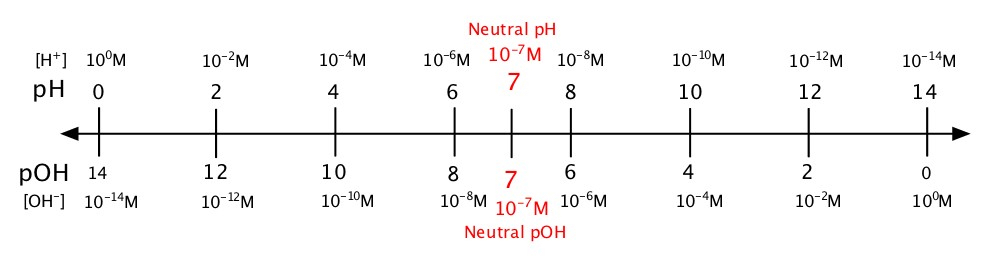

Can you see the relationship between pH and [H⁺]? (pH is only the exponent in the powers of 10! Furthermore, by looking at the equilibrium reaction of water with [H⁺] and [OH^-], that is

H₂O ⇄ H⁺ + OH^-

K_eq = K_w = 1.0 X 10^-14

The K_eq for this case has a special name, and it is called water dissociation constant K_w. If [H⁺] = 10^-7M (pH = 7), then [OH^-] is also 10^-7M! The following is the expression for K_w

K_{w} = 1.0 \times 10^{- 14} = [H^{+}] [O H^{-}]

Then, by solving for [OH^-] gives,

[O H^{-}] = \frac{K_{w}}{[H^{+}]} = \frac{1.0 \times 10^{- 14}}{1.0 \times 10^{- 7}} = 1.0 \times 10^{- 7} M

So, for water at pH=7 both [H⁺] = [OH^-] = 10^-7M.

Example: What is the pH of a solution if [H⁺] =1.5 X 10^-7M?

Since pH = -log[H⁺], we have $p H = - \log [H^{+}] = - \log [1.5 \times 10^{- 7}] = 6.8$

Example: What is the H⁺ concentration, [H⁺], if the solution pH = 5.5?

Inverse of the pH equation is [H⁺] = 10^-pH, therefore, $[H^{+}] = 10^{- p H} = 10^{- 5.5} M = 3.2 \times 10^{- 6} M$

Since pH + pOH = 14, pH = 14 - pOH, or pOH = 14 - pH, we can handle pOH. From the similar definition, $p O H = - \log [O H^{-}]$ Also, in terms of concentration, $[O H^{-}] = 10^{- p O H}$

Example: What is the H⁺ concentration, [H⁺], if the solution pOH = 5.5?

pH = 14 - pOH = 14 - 5.5 = 8.5. Then, $[H^{+}] = 10^{- p H} = 10^{- 8.5} M = 3.2 \times 10^{- 9} M$

Acid and Base Strength

Different acid (base) produces different amount of H⁺ (OH^-). Some produces a lot, and some don't. Such determination is done by using acidity constant, which is just another K_eq written for acid equilibrium.

For example of HBr(aq) or hydrobromic acid,

HBr ⇄ H⁺ + Br^-	$K_{a} = \frac{[H^{+}] [B r^{-}]}{[H B r]} = 1.0 \times 10^{9}$	pK_a = -9
CH₃CO₂H ⇄ H⁺ + CH₃CO₂^-	$K_{a} = \frac{[H^{+}] [C H_{3} C O_{2}^{-}]}{[C H_{3} C O_{2} H]} = 1.74 \times 10^{- 5}$	pK_a = 4.74

Although, K_a tells a lot, but it is more cumbersome to write, so we use pK_a.

p K_{a} = - \log K_{a}

By looking at HBr and CH₃CO₂H examples above, and we know that HBr is a strong acid, indicated by the large value of K_a, than acetic acid, we can conclude that when pK_a is small the acid is stronger. Citric acid, shown below, is polyprotic acid, meaning that there are three protons that contribute to acidity.

The pK_a of those three protons, shown in red, are:

pK_a1 = 3.13
pK_a2 = 4.76
pK_a3 = 6.39

according to Wiki.

Therefore, citric acid is more acidic than acetic acid. The second proton comes off of the citric acid and the acetic acid is about the same pH.

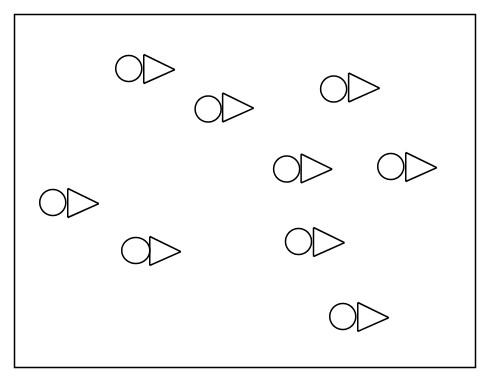
Example: Consider the initial condition below. These are the weak acid, and its K_a = 1.5. Predict the equilibrium condition.

+ Answer to equilibrium example

If you dissociate three molecules of initial condition, we have K_a = 1.5.

K_{a} = \frac{[Ο] [⊳]}{[Ο ⊳]} = \frac{[3] [3]}{[6]} = \frac{9}{6} = \frac{3}{2} = 1.5

Neutralizing Acids and Bases

When acid and base react each other, it forms water, as follows. H⁺ + OH^- → H₂O This reaction is called neutralization reaction. So, when reactions between acid and base, we use this reaction.

Titration

The reaction is generally carried out by titration where unknown concentration is determined by the volume of known concentration added.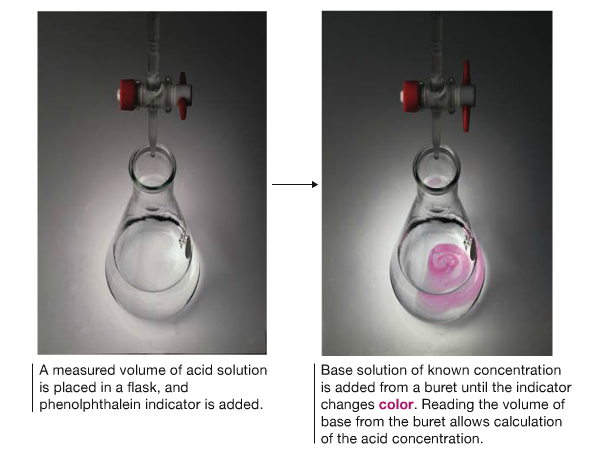

The equipment needed to perform titration includes a flask and buret. Buret is graduated such that you know how much standard solution of known concentration is added to the unknown concentration of the sample in a flask. Indicator is added to signal when enough standard solution is added to stop titration by clor change.

Here are several examples of calculations involving titration.

Example: How many mL of 2.0 M NaOH is required to titrate 20.0 mL of 1.5 M HCl?

The reaction between HCl and NaOH, you can write in two ways. One is

H⁺ + OH^- → H₂O The other one is HCl + NaOH → H₂O + NaCl I like using the first one, because it shows the essense of the reaction.

This question can be casted into dimensional analysis. Both concentrations, 2.0 M and 1.5 M, can not be the given, because they each contain mol and L (or mL). So, we start with 20.0 mL_HCl, using the first equation. $? m L_{N a O H} = 20.0 m L_{H C l} (\frac{1.5 m o l_{H C l}}{1000 m L_{H C l}}) (\frac{1 m o l_{H^{+}}}{1 m o l_{H C l}}) (\frac{1 m o l_{O H^{-}}}{1 m o l_{H^{+}}}) (\frac{1 m o l_{N a O H}}{1 m o l_{O H^{-}}}) (\frac{1000 m L_{N a O H}}{2.0 m o l_{N a O H}}) = 15 m L_{N a O H}$

Molarity

Chemical formula

⁺

Stoichiometry

Chemical formula

Molarity

These are things you know, either by inspection or given to you in the question.

If you use the second equation, the dimensional analysis is given below. See the difference? You're calculating the same quantity starting with the same number, thus you should get the same answer! $? m L_{N a O H} = 20.0 m L_{H C l} (\frac{1.5 m o l_{H C l}}{1000 m L_{H C l}}) (\frac{1 m o l_{N a O H}}{1 m o l_{H C l}}) (\frac{1000 m L_{N a O H}}{2.0 m o l_{N a O H}}) = 15 m L_{N a O H}$ Seemingly, using the first equation looks difficult, but not really. This is because for acid-base reaction is always H⁺ + OH^- → H₂O without any deviation! So, I don't have to balance the equation.

Either way of solving the problem is fine.

Example: How many g of NaOH is required to titrate 20.0 mL of 1.5 M HCl?

This time, we are looking for the g quantity of NaOH, and again starting with 20.0 mL_HCl. We're still considering the following reaction

HCl + NaOH → H₂O + NaCl So, we have

? g_{N a O H} = 20.0 m L_{H C l} (\frac{1.5 m o l_{H C l}}{1000 m L_{H C l}}) (\frac{1 m o l_{N a O H}}{1 m o l_{H C l}}) (\frac{40.0 g_{N a O H}}{1 m o l_{N a O H}}) = 1.2 g_{N a O H}

Effect of pH on Acid and Conjugate Base [ ]

In weak acid, we know from above that the acid is in equilibrium, such that

HA ⇄ H⁺ + A^-

K_{a} = \frac{[H^{+}] [A^{-}]}{[H A]}

Since pK_a = -logK_a, we can express the K_a in the following form:

p K_{a} = - \log K_{a} = - \log (\frac{[H^{+}] [A^{-}]}{[H A]}) = p H - \log \frac{[A^{-}]}{[H A]}

The logarithmic function has property such that: log (ab) = log a + log b, and log(a/b) = log a - log b This is used in the second equal sign. This is called Henderson-Husselbach equation.

Your text said(!) that an interesting relationship exists between pH and the relative concentration of [HA] and [A^-], and these are from the mathematics that

If pH = pK_a	[HA] = [A^-]
If pH < pK_a	[HA] > [A^-]
If pH > pK_a	[HA] < [A^-]

Buffers

Buffer is a capacity to resist the change in pH by adding more acid or base when pH = pK_a.

pH = pK_a happens when the concentrations of the parent acid is the same as those of conjugate base. For example,

HA ⇄ H⁺ + A^-
[HA] = [A^-] gives the buffer.

What it means is that if you want to prepare a buffer solution, often the case in biology experiment where you need to keep physiological pH (pH=7-ish) in the solution to carry out.

For example, one of more popular biological buffer is the phophate buffer system where

H₂PO₄^- ⇄ HPO₄^2- + H⁺

Example: How would you prepare 100 mL of phosphate buffer system at pH=7.0, if you have a 2.0 X 10^-2M KH₂PO₄ solution and a 2.0 X 10^-2M K₂HPO₄ solution, given that:

H₂PO₄^- ⇄ HPO₄^2- + H⁺ K_a = 6.2 X 10^-8

First, perhaps you can obtain pK_a from K_a so that whether you can actually make a buffer solution or not. $p K_{a} = - \log_{10} K_{a} = - \log_{10} (6.2 \times 10^{- 8}) = 7.21$ This is close to the desired pH. By adding 50 mL each solution, the pH of the solution becomes 7.21. You need to adjust the pH of solution by adding acid, say HCl, while monitoring the pH. Since both solutions have the same concentrations, equal volumes of two solutions should be mixed. Of course, 50.0 mL each solution is mixed.

Example: We can make buffer solution pictorially. Let's say that we have an acid in equilibrium as shown below. How many triangle anion is required to form a buffer?

+ Answer to buffer example

You need to add three more triangles to make a buffer condition, i.e. the # of acid (circle-triangle) = # of triangles = 6.

Chapter 7. Acids, Bases, and Equilibrium

Chemical Calculations!

Equilibrium Constant

Titration