Chapter 6. Thermochemistry: Chemical Energy

The Nature of Energy and Types of Energy

Energy—ability to do work or to supply heat. Mathematically,

E = q + w

where q = heat and w = work.

Heat—Thermal energy transferred from one object to another.

Another way of defining energy is by

E = T + V

where T = kinetic energy and V is the potential energy. The kinetic energy is the energy of motion for a particle with mass m, and has $T = \frac{1}{2} m v^{2}$ where v = velocity of particle. The potential energy is related to position of that particle. The functional form of potential energy differs in different systems. For example, we have seen in Chapter 7 that interaction energies between particles in H₂ molecule is given by Coulomb interaction. The distances, hence position, between two charges give the strength of the interaction.

Energy conservation: The figure below explains the relationship between energy (total energy), kinetic energy, and potential energy. Let's say that you place a ball in a symmetrical bowl-like environment without friction. The ball has a mass, m. Since we are dealing with rolling a ball, we are dealing with the potential energy in gravity which has dependence on mass and the height of the ball. The potential energy is then given by

V = V(h) = mgh

where g is the gravitational force on earth, 9.81 m/s², and h is the height of the ball compared at the bottom of the well (h = 0). Energy unit is in Joules, J = 1 kg m²/s². When you let the ball go, the position of where you put the ball, labeled with h₀ = 1, the kinetic energy is zero at the instance of letting the ball go. The ball starts to accerelate until it passes the bottom of the well, labeled h₁ = 0, at which the potential energy is zero, but kinetic energy is maximum, because of the pull of the gravity. The ball then starts to slow down until it reaches at the same height as h₀ on the opposite side, labeled h₂.

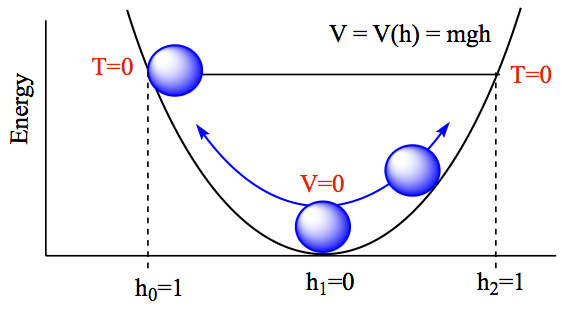
At each point of passage, the total energy is exactly the same.

h₀

₀

h₁

₁

h₀

₂

1^st Law of Thermodynamics

So, from these and in general, total energy of a process is conserved. Or total energy of the system is unchanged in a closed system.

Energy Change

Thermodynamic system: An isolated system, i.e. the system of interest such as moleucules being surrounded by surrounding, and the surrounding is enclosed by an insulated wall so that no heat, no work, no molecules escape from the surrounding. The system can exchange heat and/or work to the surrounding.

The energy of the system, internal energy which consists of molecular motions and interaction between them, can change so that the final energy can be higher or lower than its initial energy. When the final energy is higher than the initial, then the energy flows into the system from the surrounding. On the other hand, if the final energy is lower, the energy flows out of the system to the surrounding. These are depicted above.

State Function—if the quantity is independent of path, we call it state function. Energy is a state function. So, when calculating the overall energy change in the system, all you need to do, is to calculate the difference between the final energy and initial energy,

Δ E = E_final - E_initial

Introduction to Thermodynamics

We use the 1^st law of thermodynamics and develop concepts that are useful in chemistry.

The difference between the final state energy and the initial state energy, called internal energy Work: In general work is force times distance. In this chapter, we only consider work involving expansion work. Under ordinary laboratory conditions, atmospheric pressure, p, is constant. So, when expansion work is performed by the system, work done by the system is given by the following equation,

w = -p ΔV

where ΔV is the change in volume, and p is the pressure. Pressure is measured in atmosphere, atm, and the volume is measured in L with if pΔV = 1 atm L = 101 J.

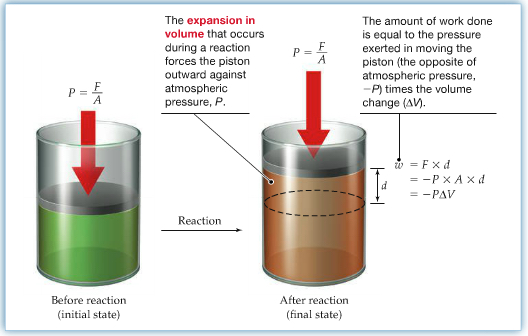
Since ΔV is defined as V_final - V_initial, if the gas is expanded, meaning that V_final > V_initial, the sign of the work is negative. In this case, it is said that the work is done by the system. If, however, the gas is compressed, then w is positive.

When the p-V work defined this way, and we can rewrite the change in the energy of the system is, ΔE = q - pΔV

Enthalpy of Chemical Rxns

We already saw that ΔE = q - pΔV. Since we can measure heat, by observing the temperatrue change, we can rearrange the equation and solve for q. Then, we get,

q = ΔE + pΔV

If there is no work, that is no change in volume, q = ΔE.

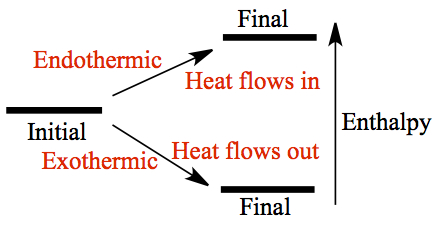
If the pressure is constant, then q = ΔE + pΔV. We define this heat measured at constant pressure to be enthalpy change, ΔH.

Enthalpy is state function, like energy. So, enthalpy change is not depended on the path. When the enthalpy of final state is higher than the initial state, it is said to be endothermic and one must inject heat into the system to achieve the final state. On the other hand, if the final state is lower in enthalpy, it is said to be exothermic where heat is generated (or liberated) by the system.

Thermodynamic Standard State

It is convenient for us to use standardized thermodynamic quantity, so that when reporting values can be compared directly.

Thermodynamic standard state—most stabel form of a substance at 1 atm pressure and at specified temperature, usually 25°C. If solution is involved, the concentration is 1 M.

We denote enthalpy change measure at the standard state to be ΔH° ← notice a knot!

Enthalpy of Physical and Chemical Change

Enthalpy change associated with physical change Melting process (fusion), and boiling (vaporization) needs extra heat to melt or vaporize. For H₂O,

H₂O (s) → H₂O (l) ΔH_fus = 6.01 kJ/mol @ 0°C

and

H₂O (l) → H₂O (g) ΔH_vap = 44.0 kJ/mol @ 100°C

Then, by the virtue of enthalpy being state function, sublimation process is

H₂O (s) → H₂O (g) ΔH_sub = ΔH_fus + ΔH_vap = 50.0 kJ/mol.

Enthalpy change associated with chemical change Of course, we are now talking about heat generated or absorbed by the reaction. We call the heat, heat of reaction. We write down the heat of reaction at the end of chemical equation, for example, combustion of propane (C₃H₈)

C₃H₈(g) + 5 O₂(g) → 3 CO₂(g) + 4 H₂O(l) ΔH° = -2220 kJ

The chemical equation that includes thermodynamic data, such as heat of reaction, is called thermochemical equation. In the above example, we have standard heat of reaction, and the reaction is exothermic.

If you reverse the reaction, that is,

3 CO₂(g) + 4 H₂O(l) → C₃H₈(g) + 5 O₂(g) ΔH° = +2220 kJ

the reaction becomes endothermic.

If on the other hand, the reaction is doubled the above,

6 CO₂(g) + 8 H₂O(l) → 2 C₃H₈(g) + 10 O₂(g) ΔH° = +4440 kJ

If halved, the value of the ΔH° must be multiplied by ½.

Example: How much heat (in kJ) is evolved or absorbed in the following reaction (not balanced) if the 4.88 g of barium hydroxide octahydrate is reacted? (Thermochemical data not affected by balancing the equation.)

Ba(OH)₂•8H₂O(s) + NH₄Cl(s) → BaCl₂(aq) + NH₃(aq) + H₂O(l) ΔH° = 80.3 kJ

The balanced equation is,

Ba(OH)₂•8H₂O(s) + 2NH₄Cl(s) → BaCl₂(aq) + 2NH₃(aq) + 10H₂O(l) ΔH° = 80.3 kJ

The rest is dimensional analysis, using 4.88g of barium hydroxide hexahyrate. $? k J = 4.88 g_{B a {(O H)}_{2} \cdot 8 H_{2} O} (\frac{1 m o l_{B a {(O H)}_{2} \cdot 8 H_{2} O}}{315.46 g_{B a {(O H)}_{2} \cdot 8 H_{2} O}}) (\frac{80.3 k J}{1 m o l_{B a {(O H)}_{2} \cdot 8 H_{2} O}}) = 1.24 k J$

A comparison of ΔH and ΔU

In general, the internal energy change and enthalpy change have the similar values. It tells you that the internal energy change is the dominant quantity of the enthalpy chnage, and the work term is relatively small. You can see that in the example below.

Example: What is the ΔU for the formation of 1 mole of CO at 1 atm and 25°C? C(graphite) + ½O₂(g) → CO(g) ΔH = -110.5 kJ/mol

Since $Δ H = Δ U + p Δ V$ The work term, according to ideal gas law, can be manipulated to $Δ H = Δ U + Δ (n R T)$ and if we assume temperature to be constant, then $Δ H = Δ U + R T Δ n$ Then, $Δ U = Δ H - R T Δ n$ Since the product produced one mole of gas, while reactant only has 0.5 mole of gas, therefore Δn in the last term is Δn = 1.0 mol - 0.5 mol = 0.5 mol.

Since the enthalpy is calculated to be -110.5 kJ/mol, $Δ U = -110.5 k J / m o l - (8.314 X 10^{-3} k J / m o l \cdot K) (298 K) \cdot (0.5 m o l) = -111.7 k J / m o l$ The work term only contributes 1.2 kJ/mol or so. It depends on the temperature, but generally the work term is quite small.

Calorimetry

Heat Capacity Since heat, q, has to do with temperature change, heat and temperature change must be proportional to each other, i.e.

q ∝ ΔT

where ΔT = T_final - T_initial. If you want an equation, instead of proportionality, we can put a proportionality constant, C, such that,

q = C ΔT

and C is called heat capacity. Heat capacity tells how much energy required by a substance to raise its temperature by 1°C. It has a unit of J/°C.

We can make heat capacity dependent on mass, it means that,

q = m c_sp ΔT

where m is the mass, and c_sp is specific heat. Specific heat is defined as energy required by 1 g of substance to raise its temperature by 1°C. Its unit is J/(°C g).

Furthermore, we can make depended on number of moles, as well. In such a case,

q = n c_m ΔT

where n is the number of moles, and c_m is the molar heat capacity, which is defined as energy required to by 1 mol of substance to raise its temperature by 1°C. Its unit is J/(°C mol).

Using these, we can measure heat.

Calorimeter—a device to measure heat. Some devices are rather elaborate, but the priciples are the same for any calorimeter. So, we illustrate a calorimetry experiment using a styrofoam coffee cup, as follows.

The styrofoam cup acts as insulating walls in the description of isolated system at the beginning of the chapter. We need to obtain temperature readings, so a thermometer is necessary, and in order to keep the temperature of water to be consistent throughout the cup, a stirring rod is provided. A calorimeter usually use water as the surrounding to the sample chamber. For simple experiments, such as the ones we perform in our labs, it doesn't have to have the sample chamber.

Specific heat of water is given as 4.182 J/(°C g).

In elaborate experiments, we can not use specific heat of water as the specific heat of surrounding, since the wall might absorb some of the heat and it might change the value. The specific heat of the calorimeter must be independently determined. For us in this course, we use the specific heat of water as the specific heat of calorimeter.

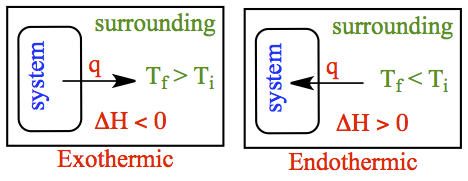
If the system releases heat to the surrounding, the surrounding receives the same amount of heat. So the amount of heat transferred is exactly the same magnitude. However, in this case, system is "pushing" the heat, and the surrounding is "pulling" the heat. This can be considered as opposite effect. In terms of mathematics, we denote this "push-pull" system to be,

q_sys = -q_cal

where q_sys is the heat from/to the system, and q_cal is the heat to/from the calorimeter (surrounding).

If we use the specific heat equation for q, then one can expand the equation above as,

m_sysc_sys ΔT_sys = -m_calc_cal ΔT_cal

The ΔT_sys on the left-hand side of the equation, is given by T_final - T_initial of the system, while ΔT_sys on the right-hand side of the equation, is given by T_final - T_initial of the calorimeter or the surrounding. In either case, T_final is common to both the system and the surrounding.

If the system is exothermic, then the heat is pushed into the surrounding. Hence the temperature of the surrounding becomes higher, compared to the initial temperature. On the other hand, if the system is endothermic, the heat is extracted from the surrounding and provided to the system. It means that the final temperature of the surrounding must become lower than the initial temperature. These situations are shown in the figure to the right.

Example: When metal beads weighing 70.50g is heated to 95.0°C, and placed into a calorimeter containing 120.0g of water initially at 23.0°C, the final temperature 30.3° is achieved. What is the specific heat, c_sp, of the metal?

In this case, the metal beads constitute a system, and the water is the surrounding. So, we can use the push-pull system of heat.

q_met = -q_sur

where q_met is the heat of metal beads, and q_sur is the heat of surrounding (or water). If we expand both sides, we get $m_{m e t} c_{s p}^{m e t} (T_{f} - T_{i}^{m e t}) = - m_{s u r} c_{s p}^{s u r} (T_{f} - T_{i}^{s u r})$ Solving for specific heat of metal beads, we get $c_{s p}^{m e t} = \frac{- m_{s u r} c_{s p}^{s u r} (T_{f} - T_{i}^{s u r})}{m_{m e t} (T_{f} - T_{i}^{m e t})} = \frac{- (120.0 g) (4.184 J / g ° C) (30.3 ° C - 23.0 ° C)}{(70.50 g) (30.3 ° C - 95.0 ° C)} = 0.803531 \frac{J}{g ° C} = 0.804 \frac{J}{g ° C}$

Another example is to solve for T_f.

Example: What is the final temperature if the following experiment is performed? Metal beads, weighing 50.0 g, heated to 100°C is placed in a calorimeter that contains 50.0 g of H₂O at 25.0°C. The specific heat of the metal is 0.385 J/g°C.

ΔT is common to both water and metal, when it reaches equilibrium. We can use the "push-pull" heat equation,

q_metal = - q_water

Expand this equation,

m_metalc_metal (T_f - T_i_{, metal}) = - m_waterc_water (T_f - T_i_{, water})

+ Here is the derivation.

m_metalc_metalT_f - m_metalc_metalT_i _{, metal} = m_waterc_water T_i_{, water} - m_waterc_waterT_f

Then, move terms containing T_f to the left-hand side, and others to the right-hand side. Then,

m_metalc_metalT_f + m_waterc_waterT_f = m_waterc_water T_i_{, water} + m_metalc_metalT_i _{, metal}

Factor out T_f on the left-hand side, you get

( m_metalc_metal + m_waterc_water ) T_f = m_waterc_water T_i_{, water} + m_metalc_metalT_i _{, metal}

Divide both sides by the content of the parenthesis, we obtain,

$T_{f} = \frac{m_{w a t e r} c_{w a t e r} T_{i, w a t e r} + m_{m e t a l} c_{m e t a l} T_{i, m e t a l}}{m_{m e t a l} c_{m e t a l} + m_{w a t e r} c_{w a t e r}}$

T_{f} = \frac{m_{w a t e r} c_{w a t e r} T_{i, w a t e r} + m_{m e t a l} c_{m e t a l} T_{i, m e t a l}}{m_{m e t a l} c_{m e t a l} + m_{w a t e r} c_{w a t e r}}

Put the numbers in, you get,

T_{f} = \frac{(50.0 g) (4.184 J / g {}^{\circ}C) (25.0 {}^{\circ}C) + (50.0 g) (0.385 J / g {}^{\circ}C) (100^{\circ} C)}{(50.0 g) (0.385 J / g^{\circ} C) + (50.0 g) (4.184 J / g {}^{\circ}C)} = {31.3}^{\circ} C

We can even apply the thermodynamic equation to ponder about the global climate change. Here is an example that opens your eyes!

Example: Climate change and its consequence. Let's say that the average annual temperature is increased by 2°C, which is set by the Paris climate accord set forth by the UN. We would like to look at the consequence of the 2°C increase in the global temperature in terms of thermodynamics of the Earth. The following calculation is a back-of-envelop type calculation, but conveys the message.

According to Ohio State University's site the specific heat of air at 300 K is 1.005 kJ/kgK. According to the Wiki page, the mass of Earth's atmosphere is 5.15 x 10¹⁸ kg, which was taken from the 1996 Edition of Handbook of Chemistry and Physics. We can calculate the energy available after 2°C increase. $q = m c Δ T = (5.15 \times 10^{18} k g) (1.005 k J / k g ° C) (2 ° C) = 1.035 \times 10^{19} k J$ What it means is that this energy is always available to the Earth, compared to today. Now, we should look at what this 10¹⁹ kJ of energy means.

We can look at, for example, the number of hurricanes we would see with the amount of energy available due to the climate change. According to NOAA, the total energy released from cloud and rain is 5.2 x 10¹⁶kJ/day. Let's say that a typical hurricane lasts 5 days. Then the total energy of one hurricane is 2.6 x 10¹⁷kJ. According to U of Arizona web site, Hurricane Katarina produced 2.4 x 10¹⁷ kJ of energy. The ratio between the energy available by the climate change and the energy of typical hurricane gives the number of hurricanes availble due to temperature rise of 2°C, and it is 38. It means that the Earth would carry, compared to today, 38 more hurricane equivalent energy always at disposal. It is quite disturbing.

Hess's Law

When multiple stpes are involved in the reaction, the sum of all enthalpy changes in different stpes give the overall enthalpy of reaction.

For example, we will look at the heat of reaction of the following.

Mg(s) + ½O₂(g) → MgO(s) ΔH° = ? Manesium burns at very high temperature and give off ultraviolet rays. So, we can not make you to do the experiment to measure the heat of reaction directly by burning it. Instead, we can do two separate experiments that are amenable to our course. We carry out the heat measurements of the two reactions that contains all species involved in the reaction above. The experiment and how to obtain ΔH_rxn is illustrated in the example below. Before we move to the example, few tips are given below, when combining two or more chemical equations.

When adding two equations We want the following equation as an overall reaction, and ultimately want the ΔH_rxn.

overall eqn

2A + 2B → 3D ΔH = ?

The two steps involved in this reaction are:

rxn 1	A + B → 3C ΔH₁
rxn 2	D → 2C ΔH₂

In rxn 1, A and B are on the correct side of the overall equation, but needs to have twice as much for both species. Thus, we need to multiply rxn 1 by 2, 2(rxn 1). In order to have D on the right-hand side in the overall equation, we must flip rxn 2 equation around, and make 2C → D, at the same time mutiply by 3 to have the correct number of D. When equation is flipped, you're reversing the flow of energy (heat), so the sign of the ΔH must also be flipped. Therefore, the enthalpy of reaction 2 is now -ΔH₂.

In addition, there is no C in the overall equation, therefore, we must have the same number of C in both reactions. It means that we need to multiply 2 to the first reaction, and 3 to the second. Then, we have,

2(rxn 1)	2A + 2B → 6C 2ΔH₁
-3(rxn 2)	6C → 3D -3ΔH₂

overall 2A + 2B → 3D ΔH = 2ΔH₁ - 3ΔH₂

Here is an example of experiment we actually do in our lab dealing with the Hess's law.

Example: Calculate the heat of formation of MgO(s), i.e., Mg(s) + ½O₂(g) → MgO(s), from experimental data given in the table below. Two experiments are performed (as you would do in the experiment next week!). One is to dissolve Mg(s) by hydrochloric acid, and measure the heat of the reaction. The second experiment is to dissolve MgO(s) in hydrochloric acid, and measure the heat. These two reactions are given below. Rxn 1 Mg(s) + 2HCl(aq) → MgCl₂(aq) + H₂(g) ΔH₁ = ?

Rxn 2 MgO(s) + 2HCl(aq) → MgCl₂(aq) + H₂O(l) ΔH₂ = ?

In addition to above two reactions, we need the heat of formation of water to be able to make the overall equation to be correct.

2H₂(g) + O₂ → 2H₂O(l) ΔH = -571.7 kJ/mol

Say that the following is the result of two reactions:

	mass	Volume	T_initial	T_final
Rxn 1	0.202g (Mg)	100 mL HCl	21.7°C	31.7°C
Rxn 2	1.040g (MgO)	100 mL HCl	22.1°C	30.2°C

The concentration of HCl used in both cases is 2.87 M, and its specific heat is 3.47 J/g°C. The density of HCl(aq) at the concentration is 1.048 g/mL.

Heat of reaction 1 is calculated by by using,

q_cal = m c ΔT = (104.8g)(3.47 J/g°C) (31.7°C - 21.7°C) = 3636.56 J Here the mass of the HCl is obtained from the density (1.048 g/mL).

Similar calculation for rxn 2 yields q_cal = 2945.61 J. Therefore, q_{rxn 1} = - q_cal = -3636.56 J, and q_{rxn 2} = - 2945.61 J.

Now we need to know the number of moles of Mg or MgO in the respective solution so that q is converted to ΔH, and they are, $\begin{array}{l} ? m o l_{M g} = 0.202 g_{M g} (\frac{1 m o l_{M g}}{24.3050 g_{M g}}) = 8.311 \times 10^{- 3} m o l_{M g} \\ ? m o l_{M g O} = 1.040 g_{M g O} (\frac{1 m o l_{M g O}}{40.3044 g_{M g O}}) = 2.580 \times 10^{- 2} m o l_{M g O} \end{array}$

Dividing the q_{rxn 1} by the number of moles of Mg, ΔH_{rxn 1} = -437.6 kJ/mol(Mg). Similarly, ΔH_{rxn 2} = -114.7 kJ/mol(MgO).

Now we have to rearrange Rxn 1, Rxn 2, as well as the heat of formation of H₂O to obtain the equation of interest.

Rxn 1 stays the same since Mg appears on the reactant side in rxn 1 as well as in the overall equation. However, rxn 2 needs to be reversed since MgO appears in the product side of the overall equation, meaning that multiply the rxn 2 equation by -1. Furthermore, we need to cancel H₂ in rxn 1, therefore, we need to divide the heats of formation of water by 2.

Rxn 1 Mg(s) + ~~2HCl(aq)~~ → ~~MgCl₂(aq)~~ + ~~H₂(g)~~ ΔH₁ = -437.6 kJ/mol

Rxn 2 ~~MgCl₂(aq)~~ + ~~H₂O(l)~~ → MgO(s) + ~~2HCl(aq)~~ ΔH₂ = +114.7 kJ/mol

ΔH_f ~~H₂(g)~~ + ½O₂(g) → ~~H₂O(l)~~ ΔH_f = -285.8 kJ/mol

overall Mg(s) + ½O₂(g) → MgO(s) ΔH_overall = ΔH₁ + ΔH₂ + ΔH_f = -608.7 kJ/mol

The enthalpy of formation of MgO is calculated to be -608.7 kJ/mol, which is exothermic with large magnitude.

Hess's law can be illustrated in terms of energy diagram. For a reaction,

Overall CH₃CH₂OH(l) + O₂(g) → CH₃CO₂H(l) + H₂O(l) ΔH°_rxn = ???

We have the reaction in two steps:`

Rxn 1 CH₃CH₂OH(l) + ½O₂(g) → CH₃CHO(g) + H₂O ΔH° = -174.2 kJ

Rxn 2 CH₃CHO(g) + ½O₂(g) → CH₃CO₂H(l) ΔH° = -318.4 kJ

Standard Heats of Formation

Formation Reaction—reaction to make a compound from its stable elements. The reactants are the elements and the product is the moleucle of interest. For example, the formation reaction for water is,

H₂(g) + ½O₂ → H₂O(l)

as making liquid water from its elements, hydrogen molecule and oxygen molecule. The enthalpy associated with the formation reaction is called, heat of formation, ΔH_f . As we have seen already,

H₂(g) + ½O₂ → H₂O(l) ΔH_f(H₂O) = -285.8 kJ/mol

If we measure the heat of formation at standard state, then the heat of formation is standard heat of formtion, ΔH°_f.

Heats of Formation of Elements are zero! So, H₂, O₂, C(graphite), Cu, Fe, Cl₂ have all zero for its formation enthalpy.

Standard Reaction Enthalpy. What's useful about the heats of formation, is the fact that one can obtain heat of reaction, by the following for the reaction,

aA + bB → cC + dD

where small letters, a, b, c, and d are the stoichiometry of the balanced chemical equation. Then,

$Δ H_{r x n}^{\circ} = c Δ H_{f}^{\circ} (C) + d Δ H_{f}^{\circ} (D) - a Δ H_{f}^{\circ} (A) - b Δ H_{f}^{\circ} (B)$ This is quite useful because you don't have to know anything about the reaction to obtain the enthalpy of the reaction, simply by looking up on a table of ΔH°_f (In our case, look at Appendix B in our text).

Example: At 850°C, CaCO₃ undergoes substatial decomposition to yield CaO and CO₂. Assuming that ΔH°_f values are the same at 850° as they are at 25°, calculate the enthalpy change (in kJ) if 66.8 g of CO₂ are produced in one reaction.

Chemical equation associated with the question is:

CaCO₃ → CaO + CO₂

According to Table 6.4 of the textbook, ΔH°_f(CaCO₃) = -1206.9 kJ/mol, ΔH°_f(CaO) = -635.6 kJ/mol, and ΔH°_f(CO₂) = -393.5 kJ/mol. Then, the $Δ H_{r x n}^{\circ}$ is given by, $Δ H_{r x n}^{\circ} = Δ H_{f}^{\circ} (C a O) + Δ H_{f}^{\circ} (C O_{2}) - Δ H_{f}^{\circ} (C a C O_{3}) = - 635.6 - 393.5 + 1206.9 k J / m o l = 177.8 k J / m o l$

Heat of Solution and Dilution

Heat of Solution

Heat being absorbed or released by the solute upon dissolving to make a solution is called, enthalpy of solution, ΔH_sol'n

In the figure above, the heat associated with dissolving solute into a solution is explained in terms of three step process. The first step is to have the crystal (or any other solid) with blue circles is separated into individual molecules. Since there is attractive force exists between solute molecues, the process of separation is endothermic, labeled as ΔH_solute. The solvent must make space for solute molecules to be embedded into the solvent, so the heat needed to remove some of the solvent molecules to make space for solute molecules again held by attractive forces, is the ΔH_solv. The heat associated with the interaction of solute and solvent molecules are labeled as ΔH_intxn. The overall process, ΔH_sol'n, is the blue arrow is the exothermic, in this particular case.

So, in order to dissolve some solute into some solvent we need to consider three factors, ΔH_solute, ΔH_solv, and ΔH_intxn.

ΔH_sol'n = ΔH_solute + ΔH_solv + ΔH_intxn